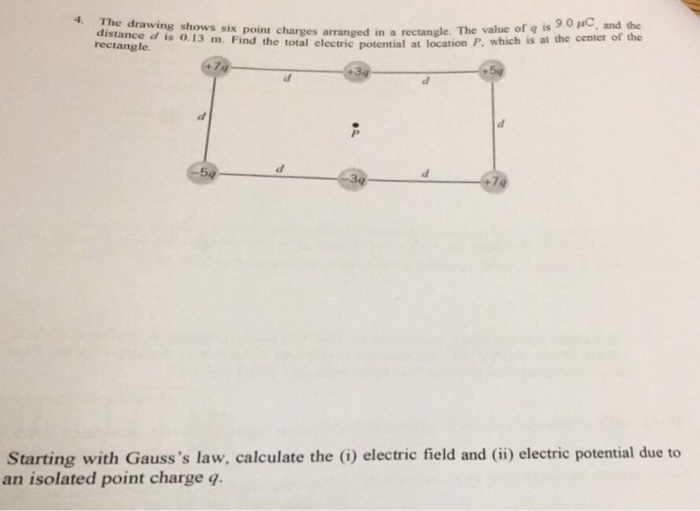
The Drawing Shows Si Point Charges Arranged In A Rectangle
The Drawing Shows Si Point Charges Arranged In A Rectangle - The value of q is 9.0 μc, and the distance d is 0.13 m. Web the drawing shows six point charges arranged in a rectangle. Web the drawing shows six point charges arranged in a rectangle. The drawing shows six point charges arranged in a rectangle. The value of q q is 9.0μc 9.0 μ c, and the distance d d is 0.13m 0.13 m. Find the total electric potential at location p, which is at the center of the rectangle. Chapter 19, problem 19 the drawing shows six point charges arranged in a rectangle. Electric potential = ʃ (kq/r) the attempt at a solution. Calculate the electric potential due to each individual point charge at location p using the formula $v = \frac {kq} {r}$, where $k$ is the electrostatic constant ($8.99 \times 10^9 \, \mathrm {n \cdot m^2/c^2}$), $q$ is the charge, and $r$ is the distance between the charge and location p. Here’s the best way to solve it.
The drawing shows six point charges arranged in a rectangle. Find the total electric potential at location p, which is at the center of the rectangle. The value of q is 9.0 μc, and the distance d is 0.13 m. The value of q is 8.08 μc, and the distance d is 0.247 m. The value of q is 8.04 μc, and the distance d is 0.365 m. The value ofq is 9.0 µc, and the distance. The drawing shows six point charges arranged in a rectangle.
Chapter 19, problem 19 the drawing shows six point charges arranged in a rectangle. The value of g is 9.0 pc, and the distance d is 0.13 m. Here’s the best way to solve it. Find the total electric potential at location p, which is at the center of the rectangle. Find the total electric potential at location p p, which is at the center of the rectangle.
SOLVED The drawing shows six point charges arranged in a rectangle
Solved The drawing shows six point charges arranged in a
Solved The Drawing Shows Six Point Charges Arranged In A
Solved Four Point Charges Are Arranged In A Rectangle Of
The drawing shows six point charges arranged in a rectangle. The value
Solved The drawing shows six point charges arranged in a
Physics Help! Help The drawing shows six point charges arranged in a
SOLVED The drawing shows six point charges arranged in a rectangle
The value of q is 9.0 μc, and the distance d is 0.13 m. Find the total electric potential at location p, which is at the center of the rectangle. The value of q is 9.60 \muc, and the distance d is 0.22 m. Find the total electric potential at location p, which is at the center of the rectangle. Find the total electric potential at location p, which is at the center of the rectangle. The value of q is 8.08 μc, and the distance d is 0.247 m.
Since p is at the center of the rectangle, the distances from p to each of the four corner charges are equal. Foreignlove5860 is waiting for your help. Find the total electric potential at location p, which is at the center of the rectangle. The value of $q$ is $9.0 \mu \mathrm{c}$, and the distance $d$ is $0.13 \mathrm{m}$. Find the total electric potential at location p, which is at the center of the rectangle.
The value of g is 9.0 pc, and the distance d is 0.13 m. The value of q is 3.87 µc, and the distance d is 0.274 m. Web the drawing shows six point charges arranged in a rectangle. The value of q is 8.08 μc, and the distance d is 0.247 m.
Foreignlove5860 Is Waiting For Your Help.
The value of q is 9.0 μc, and the distance d is 0.13 m. The value of q is 6.58 μc, and the distance d is 0.196 m. Web physics questions and answers. The value of g is 9.0 pc, and the distance d is 0.13 m.
The Value Of Q Is 5.01 Μc, And The Distance D Is 0.205 M.
Find the total electric potential at location p p, which is at the center of the rectangle. The drawing shows six point charges arranged in a rectangle. Find the total electric potential at location p p, which is at the center of the rectangle. The value of q is 8.30 μ c, and the distance d is 0.468 m.
First, We Need To Find The Distance From Each Charge To Point P.
The drawing shows six point charges arranged in a rectangle. Find the total electric potential at location $p,$ which is at the center of the rectangle. Web the drawing shows six point charges arranged in a rectangle. The value of q is 8.31 µc, and the distance d is 0.18 m.
Click The Card To Flip 👆.
Find the total electric potential at location p, which is at the center of the rectangle. Electric potential = ʃ (kq/r) the attempt at a solution. Web the drawing shows six point charges arranged in a rectangle. The value of q is 9.60 \muc, and the distance d is 0.22 m.