Conservative Vector Field E Ample

SOLVED 2324 Is the vector field shown in the figure conservative

We then develop several equivalent properties shared by all conservative vector fields. The aim of this chapter is to study a class of vector fields over which line integrals are independent of the particular path..

Multivariable Calculus Conservative vector fields. YouTube

Web the curl of a vector field is a vector field. 26.2 path independence de nition suppose f : The aim of this chapter is to study a class of vector fields over which line.

PPT VECTOR CALCULUS PowerPoint Presentation, free download ID3194892

Then if p p and q q have continuous first order partial derivatives in d d and. 17.3.2 test for conservative vector fields. A vector field with a simply connected domain is conservative if and.

Conservative Vector at Collection of Conservative

Web a conservative vector field is a vector field that is the gradient of some function, say \vec {f} f = ∇f. 17.3.1 types of curves and regions. Web a vector field f ( x,.

Conservative Vector at Collection of Conservative

Web we also show how to test whether a given vector field is conservative, and determine how to build a potential function for a vector field known to be conservative. Gravitational and electric fields are.

Conservative Vector Field YouTube

Web we examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals of conservative vector fields. Web but if \(\frac{\partial f_1}{\partial y} = \frac{\partial.

Solved 4. Conservative Vector Fields. Consider the vector

Web explain how to find a potential function for a conservative vector field. This scalar function is referred to as the potential function or potential energy function associated with the vector field. In this case,.

PPT VECTOR CALCULUS PowerPoint Presentation, free download ID5567505

The 1st part is easy to show. In fact there are fields that are not conservative but do obey \(\frac{\partial f_1}{\partial y}=\frac{\partial f_2}{\partial x}\text{.}\) we'll see one in example 2.3.14, below. Web conservative vector fields.

∫c(x2 − zey)dx + (y3 − xzey)dy + (z4 − xey)dz ∫ c ( x 2 − z e y) d x + ( y 3 − x z e y) d y + ( z 4 − x e y) d z. The vector field →f f → is conservative. In fact there are fields that are not conservative but do obey \(\frac{\partial f_1}{\partial y}=\frac{\partial f_2}{\partial x}\text{.}\) we'll see one in example 2.3.14, below. 17.3.1 types of curves and regions. This scalar function is referred to as the potential function or potential energy function associated with the vector field. Explain how to test a vector field to determine whether it is conservative.

Web a vector field f ( x, y) is called a conservative vector field if it satisfies any one of the following three properties (all of which are defined within the article): 8.1 gradient vector fields and potentials. Use the fundamental theorem for line integrals to evaluate a line integral in a vector field. Web for a conservative vector field , f →, so that ∇ f = f → for some scalar function , f, then for the smooth curve c given by , r → ( t), , a ≤ t ≤ b, (6.3.1) (6.3.1) ∫ c f → ⋅ d r → = ∫ c ∇ f ⋅ d r → = f ( r → ( b)) − f ( r → ( a)) = [ f ( r → ( t))] a b. First, find a potential function f for f and, second, calculate f(p1) − f(p0), where p1 is the endpoint of c and p0 is the starting point.

This scalar function is referred to as the potential function or potential energy function associated with the vector field. Explain how to test a vector field to determine whether it is conservative. The test is followed by a procedure to find a potential function for a conservative field. Here is a set of practice problems to accompany the conservative vector fields section of the line integrals chapter of the notes for paul dawkins calculus iii course at lamar university.

Depend On The Specific Path C C Takes?

In this case, we can simplify the evaluation of \int_ {c} \vec {f}dr ∫ c f dr. Rn!rn is a continuous vector eld. Web example the vector eld f(x;y;z) = 1 (x2 + y2 + z 2 2)3 (x;y;z) is a conservative vector eld with potential f(x;y;z) = p 1 x2 + y2 + z2: Gravitational and electric fields are examples of such vector fields.

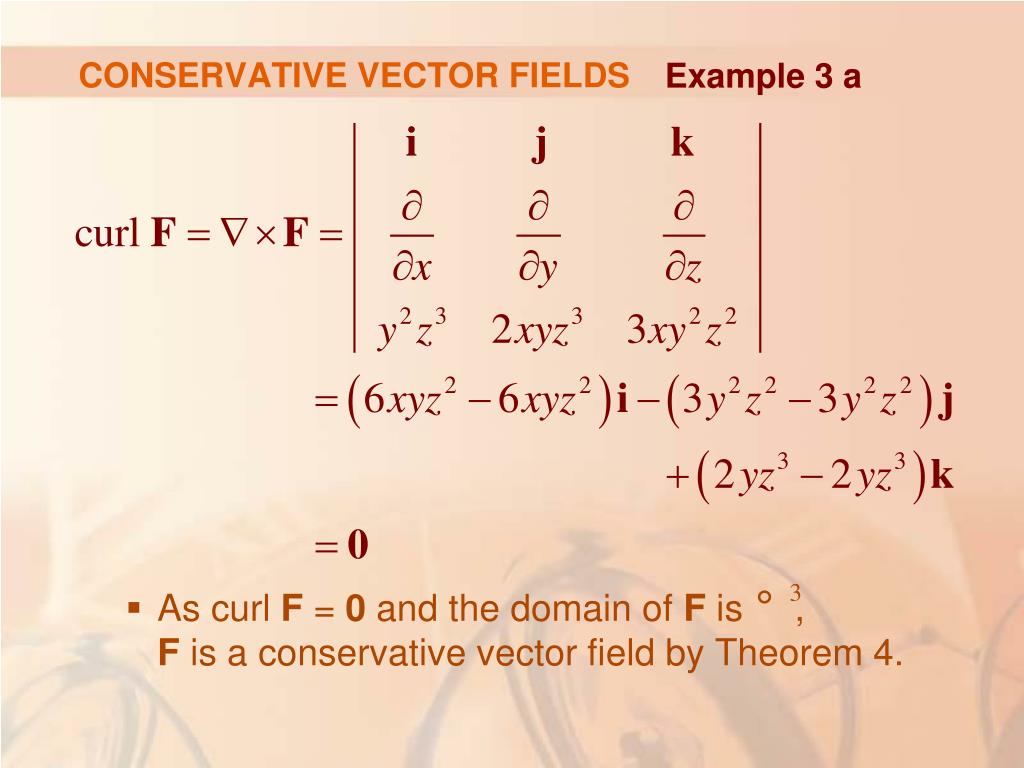
Web But If \(\Frac{\Partial F_1}{\Partial Y} = \Frac{\Partial F_2}{\Partial X}\) Theorem 2.3.9 Does Not Guarantee That \(\Vf\) Is Conservative.

Web in vector calculus, a conservative vector field is a vector field that is the gradient of some function. ∂p ∂y = ∂q ∂x ∂ p ∂ y = ∂ q ∂ x. The integral is independent of the path that $\dlc$ takes going from its starting point to its ending point. Before continuing our study of conservative vector fields, we need some geometric definitions.

In Fact There Are Fields That Are Not Conservative But Do Obey \(\Frac{\Partial F_1}{\Partial Y}=\Frac{\Partial F_2}{\Partial X}\Text{.}\) We'll See One In Example 2.3.14, Below.

The test is followed by a procedure to find a potential function for a conservative field. 8.1 gradient vector fields and potentials. The curl of a vector field at point \(p\) measures the tendency of particles at \(p\) to rotate about the axis that points in the direction of the curl at \(p\). 17.3.1 types of curves and regions.

We Then Develop Several Equivalent Properties Shared By All Conservative Vector Fields.

The 1st part is easy to show. 26.2 path independence de nition suppose f : Web we examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals of conservative vector fields. Use the fundamental theorem for line integrals to evaluate a line integral in a vector field.